
- Stein And Shakarchi Complex Analysis Chapter 1 Solutions
- Stein And Shakarchi Complex Analysis Manual Solutions
- Complex Analysis Stein Answer
- Stein And Shakarchi Complex Analysis
- Stein And Shakarchi Complex Analysis Chapter 3 Solutions
*Solution Manual of Elias M.Stein, Rami Shakarchi:
ex1:————————————————–
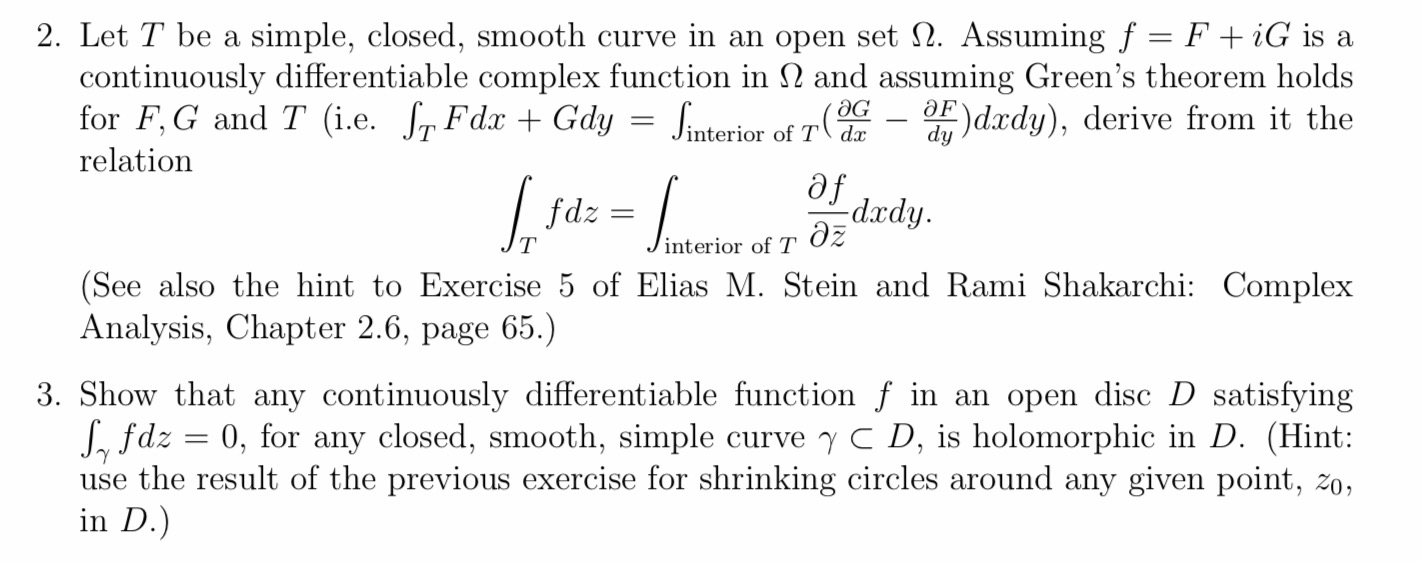
And the textbook is Complex Analysis by Stein and Shakarchi (ISBN13: 978-0-691-11385-2). Note to students: it’s nice to include the statement of the problems, but I leave that up to you. Stein and Shakarchi move from an introduction addressing Fourier series and integrals to in-depth considerations of complex analysis; measure and integration theory, and Hilbert spaces; and, finally, further topics such as functional analysis, distributions and elements of probability theory.
please check 2012f_Lebesgue-integrals_Lecture-note
also you can take a look at these proofs:
ex2:————————————————–
part1: exercise2
part2:exercise2
ex3:————————————————–
also you can use Corollary 1.2 in 2012f_Lebesgue-integrals_Lecture-note.
for the second part try to use Theorem 1.8 in 2012f_Lebesgue-integrals_Lecture-note.
ex4:————————————————–
part1:exercise4p1
Stein And Shakarchi Complex Analysis Chapter 1 Solutions
for second and third part check Solution Manual of Elias M.Stein, Rami Shakarchi page 4
ex5:————————————————–
ex6:————————————————–
hint:
ex8:————————————————–


similar to part Solution Manual of Elias M.Stein, Rami Shakarchi page 7
Stein And Shakarchi Complex Analysis Manual Solutions
ex14:————————————————–
ex15:————————————————–
Complex Analysis Stein Answer
ex16:————————————————–
Stein And Shakarchi Complex Analysis

Stein And Shakarchi Complex Analysis Chapter 3 Solutions
check Solution Manual of Elias M.Stein, Rami Shakarchi page 9